高圧ダイカスト マグネシウム-アルミニウム基合金の機械的特性と変形挙動

1. 概要:

  • タイトル: 高圧ダイカスト マグネシウム-アルミニウム基合金の機械的特性と変形挙動 (Mechanical Properties and Deformation Behaviour of High-Pressure Die-Cast Magnesium-Aluminium Based Alloys)
  • 著者: Hua Qian Ang (Vivian)
  • 発表年: 2017年10月
  • 掲載誌/学会: RMIT大学 工学部 工学・健康科学大学院 博士論文
  • キーワード: 高圧ダイカスト、マグネシウム合金、変形挙動、機械的特性、ひずみ速度感受性、構成モデル
Fig. 1 Pictures of one of the better castings a and c die temperature 250 °C and injection velocity 2.0 m/s, and worst castings b and d die temperature 180 °C and injection velocity 1.3 m/s for AS31
Fig. 1 Pictures of one of the better castings a and c die temperature 250 °C and injection velocity 2.0 m/s, and worst castings b and d die temperature 180 °C and injection velocity 1.3 m/s for AS31

2. 研究背景:

  • 研究トピックの社会的/学術的背景: 燃料効率の向上と温室効果ガス排出量の削減に対する要求の高まりから、自動車用途における軽量化の必要性が生じています。高圧ダイカスト マグネシウム合金は、低密度と高い比強度により、自動車構造部品への応用が期待されており、アルミニウム、鋼、鋳鉄に代わる軽量化の有力な候補です。構造用合金には、衝突時やセルフピアッシングリベットなどの製造プロセスにおけるエネルギー吸収のために、高い強度と延性が求められます。
  • 既存研究の限界: マグネシウム合金の変形において、弾性や塑性に比べて、アネラスティック性（非弾性）は注目されていません。既存研究では、アネラスティック性に対するひずみ速度やアルミニウム含有量の影響に関する包括的な調査が不足しています。
  • 研究の必要性: 構造用途における高圧ダイカスト マグネシウム合金の機械的特性を向上させるためには、荷重下での変形挙動の基礎的な理解が不可欠です。本研究は、これらの合金の変形メカニズムを解明することを目的としています。

3. 研究目的と研究課題:

  • 研究目的: 本研究の主な目的は、市販の高圧ダイカスト マグネシウム-アルミニウム基合金、具体的には Mg-4Al-0.3Mn (AM40)、Mg-6Al-0.3Mn (AM60)、Mg-9Al-1Zn (AZ91)、Mg-4Al-4RE (AE44) の機械的特性と変形挙動を、10-6～10-1 s-1 の広範囲なひずみ速度範囲で調査することです。
  • 主な研究課題:
    • 高圧ダイカスト マグネシウム-アルミニウム基合金の機械的特性と変形挙動は、ひずみ速度とアルミニウム含有量によってどのように影響を受けるか？
    • マグネシウム合金の耐力測定において、アネラスティック性はどのような影響を与えるか？
    • 全体的な変形挙動に対する弾性、アネラスティック性、塑性の寄与はそれぞれ何か？また、それらはひずみ速度とアルミニウム含有量によってどのように依存するか？
    • 変形メカニズム（すべり、双晶）は、応力-ひずみ曲線の異なる段階とどのように関連付けられるか？また、これらの段階をモデル化するための構成式を開発できるか？
  • 研究仮説:
    • アネラスティック性は、マグネシウム合金の耐力測定に曖昧さをもたらす。
    • 高圧ダイカスト マグネシウム-アルミニウム基合金のひずみ速度感受性は、α-マグネシウムマトリックス中のアルミニウム固溶体含有量の増加とともに減少する。
    • アネラスティック性はひずみ速度に鈍感であり、一方、 プリズムすべりによる塑性はひずみ速度に敏感である。

4. 研究方法:

  • 研究デザイン: 本研究では、標準化された耐力測定法の批判的レビューと、一連の実験的研究を含む実験的デザインを採用しました。これらの研究は、以下に焦点を当てています。
    • ダイカスト マグネシウム合金の耐力測定
    • ひずみ速度とアルミニウム含有量が変形挙動に及ぼす影響
    • さまざまなひずみ速度下でのダイカスト マグネシウム-アルミニウム基合金のアネラスティック性
    • 変形挙動の構成モデリング
  • データ収集方法: データは、以下を通じて収集されました。
    • 広範囲なひずみ速度 (10-6～10-1 s-1) にわたる単調引張試験
    • アネラスティック性を特徴づけるための繰返し引張荷重-除荷試験
    • 4つの標準化された耐力測定法（Method 1、Method 2、Method 3、Method 4）の適用
  • 分析方法: 収集されたデータは、以下を用いて分析されました。
    • 機械的特性の統計的および定性的な分析
    • 変形段階とパラメータを特定するための応力-ひずみ曲線分析
    • 変形挙動の予測モデルを開発するための構成モデリング
    • 走査型電子顕微鏡 (SEM)、エネルギー分散型X線分光法 (EDX)、電子後方散乱回折法 (EBSD) を用いた微細構造特性評価
  • 研究対象と範囲: 研究は、市販の高圧ダイカスト マグネシウム-アルミニウム基合金に焦点を当てました。
    • Mg-4Al-0.3Mn (AM40)
    • Mg-6Al-0.3Mn (AM60)
    • Mg-9Al-1Zn (AZ91)
    • Mg-4Al-4RE (AE44)
    • T5処理 AE44

5. 主な研究成果:

  • 主な研究成果:
    • 耐力測定: アネラスティック性は、標準化された方法を用いた耐力測定に矛盾を引き起こすことが判明しました。高圧ダイカスト マグネシウム合金の耐力測定において、より正確な 0.2% 残留塑性ひずみを達成するために、より高いオフセットひずみ 0.45% を用いる実用的なアプローチが提案されました。
    • ひずみ速度感受性: 固溶体中のアルミニウム含有量が少ない合金は、より高いひずみ速度感受性を示し、加工硬化と引張/降伏比の増加として現れました。アルミニウム固溶体含有量の増加に伴うひずみ速度感受性の低下は、α-マグネシウムマトリックス内の動的ひずみ時効に起因すると考えられました。 プリズムすべりと 錐面すべりの高いひずみ速度感受性も観察されました。
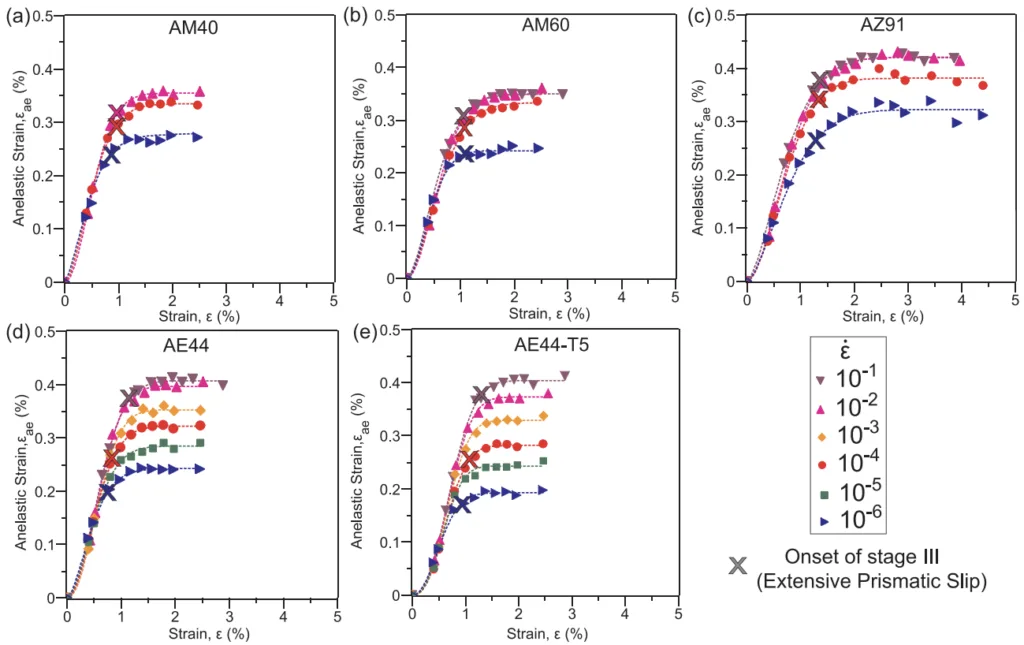
    • アネラスティック性: アネラスティック性はひずみ速度に鈍感であることが判明し、一方、 プリズムすべりによる塑性はひずみ速度に敏感でした。 プリズムすべりの高いひずみ速度感受性は、ひずみ速度による最大アネラスティック性の変動につながりました。固溶体中のアルミニウム含有量が最も多い AZ91 は、AM40 や AM60 と比較して、最大の最大アネラスティック性を示しました。
    • 構成モデリング: 半経験的モデルが開発され、ほとんどの合金の実験的な応力-ひずみ曲線によく適合しましたが、AZ91 の第 II-III 遷移領域では応力をわずかに過大評価しました。
  • 統計的/定性的な分析結果:
    • Method 1 による耐力測定は、弾性率 E に依存し、応力増加とともに 45 GPa から 38 GPa に減少します。
    • 割線弾性率 Esec は、塑性ひずみと繰返し試験におけるループ幅の増加とともに減少します。
    • アネラスティックひずみは、試験した合金の 0.2% 永久塑性ひずみで約 0.25% です。
    • ひずみ速度感受性 m は、アルミニウム含有量の増加とともに急速に減少し、Al 含有量が 3 wt.% を超えると無視できるようになります。
    • 第 III 段階における加工硬化率 Θ は、合金組成に応じて 1～2 GPa の範囲で変化します。
  • データ解釈: ダイカスト マグネシウム合金の変形挙動は複雑であり、弾性、アネラスティック性、塑性の 3 つの変形領域に分離できます。さらに分解すると、応力-ひずみ曲線には、弾性 (第 I 段階)、 底面すべりと双晶 (第 II 段階)、 プリズムすべり (第 III 段階)、 錐面すべり (第 IV 段階) の 4 つの段階があることが明らかになりました。
  • 図表リスト:
    • Figure 2.14: An overview of the cyclic tension loading-unloading stress-strain curve of pure magnesium
    • Figure 2.17: Anelastic strain (%) at 2% of plastic strain of pure magnesium, AZ91, Mg-0.8Zn and Mg-2.3Zn and Mg-1.5Gd as a function of grain size.
    • Figure 2.18: The anelastic strain as a function of the applied tensile (solid lines) or compressive (dashed lines) plastic strain, for AZ91 in two different casting conditions: sand-cast (sc) and high-pressure die-cast (hpdc)
    • Figure 2.19: Anelastic strain as a function of the tensile (solid lines) and compressive (dashed lines) plastic strain, for pure magnesium and various (a) zinc contents, (b) gadolinium contents and (c) aluminium contents.
    • Figure 2.20: Stress-strain curves of HPDC AS21 with a best fit model of stress, σ as a function of strain, ε and strain rate, ε̇.
    • Figure 2.21: Strain-rate sensitivity, m versus aluminium content (wt.%) for pure magnesium, and various ternary magnesium alloys.
    • Figure 2.22: Strain-rate sensitivity, m as a function of strain rates covering from creep to dynamic strain rates, for pure magnesium and other ternary magnesium alloys.
    • Figure 3.14: Room-temperature monotonic tensile curves of die-cast magnesium alloys AM40, AM60, AZ91, AE44 and T5-aged AE44 at strain rate, ε̇ of 10-4 s-1
    • Figure 3.15: A comparison of monotonic and cyclic tensile curves of AM60 at strain rate 10-3 s-1
    • Figure 1: Proof stress measurement methods: (a) Method 1, (b) Method 2 as specified in ASTM E8M-09 and (c) Method 3 and (d) Method 4 as stated in ISO 6892-1
    • Figure 2: Proof stress values measurement by Method 1 for die-cast AE44, AM60 and AZ91 alloys
    • Figure 3: Tensile curves of die-cast AE44, AM60, AZ91 and A380 showing respectively proof stress values measured by Method 2
    • Figure 4: Cyclic stress-strain curve of AE44 showing proof stress measured by Method 3
    • Figure 5: Cyclic stress-strain curve of A380 showing proof stress measurement by Method 3
    • Figure 6: (a) Secant elastic modulus, Esec (GPa) and (b) anelastic strain (%) as a function of tensile permanent plastic strain (%)
    • Figure 7: Proof stress measurement by Method 4 for AE44 where the alternative elastic modulus, Ealt is 33.3 GPa
    • Figure 8: Inconsistency within Method 4 providing different alternative elastic moduli, Ealt, leading to a variation in proof stress values
    • Figure 9: An overview of monotonic and cyclic stress-strain curves of die-cast (a) AE44, (b) AM60 and (c) AZ91
    • Figure 10: Permanent plastic strain as a function of offset strain (plastic strain + anelastic strain) for die-cast magnesium alloys.
    • Figure 11: Stress-strain curve ofAM60 showing a comparison of 0.2% and 0.5% offsets and the influence of errors in modulus determination
    • Figure 1: True stress-strain curves of as-cast (a) AM40, (b) AM60, (c) AZ91, (d) AE44 and T5-aged (e) AE44 at different nominal strain rates, ε̇
    • Figure 2: Effects of strain rate on (a) strength (MPa) and (b) elongation to fracture (%) of as-cast AM40, AM60, AZ91, AE44 and T5-aged AE44
    • Figure 3: Variations of strain-rate sensitivity with (a) true strain, covering strain rates, ε̇ from 10-6-10-1 s-1 and (b) with strain rate at 3% strain.
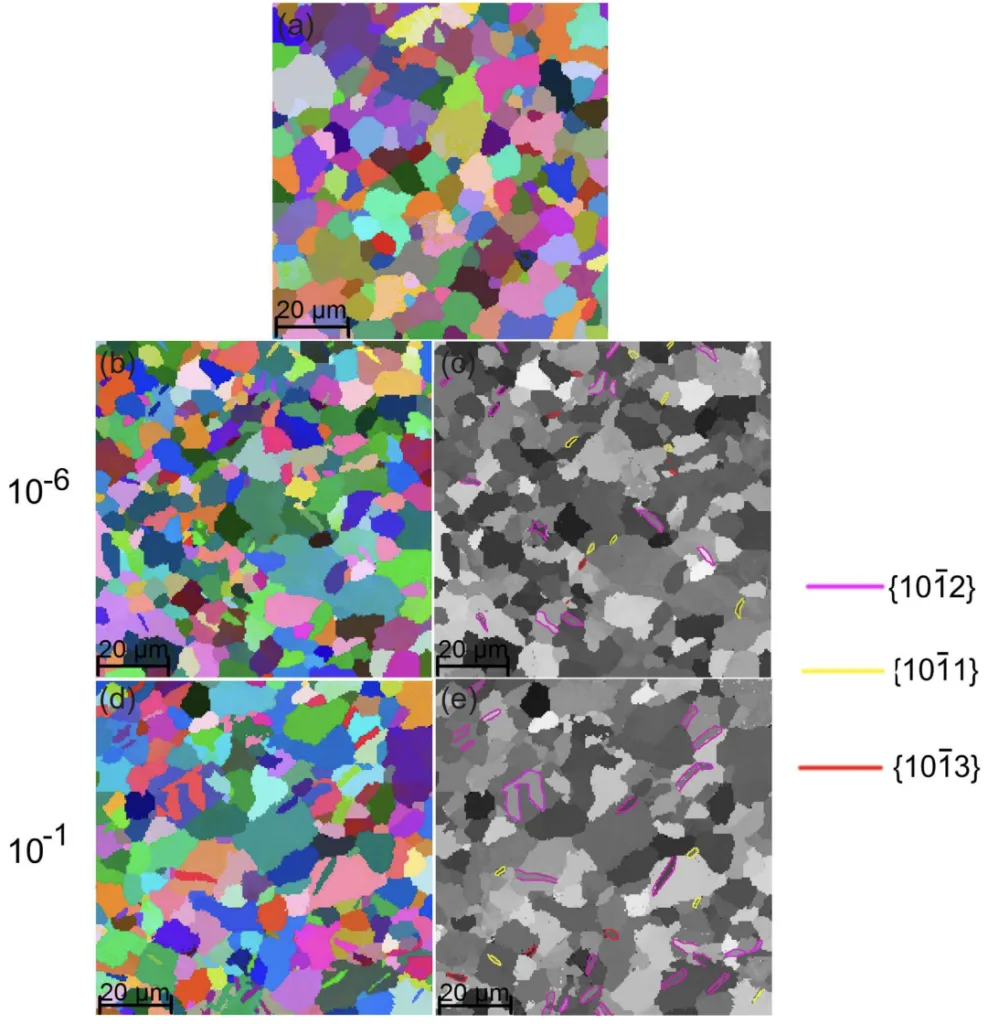
    • Figure 4: EBSD maps showing formation of different types of twins in as-cast AE44, AM60, and AZ91 specimens after tensile testing at different strain rates.
    • Figure 5: Secondary electron images of fracture surfaces of (a, b) AM60, (c, d) AZ91 and (e, f) AE44 at different strain rates
    • Figure 6: Backscattered electron images and EDX line profiles of the α-Mg matrix in (a) AM40, (b) AM60, (c) AZ91, (d) AE44, and (e) AE44-T5.
    • Figure 7: A correlation of strain-rate sensitivity and Al solute concentration: (a) Inverse strain-rate sensitivity versus Al solute level in Mg phase and (b) strain-rate sensitivity as a function of sample diameter and thicknesses in die-cast AM60.
    • Figure 1: An overview of cyclic stress-strain curve of die-cast AE44 at 10-4 s-1
    • Figure 2: Monotonic flow curves of (a) AZ91 and (b) AE44 at strain rates 10-6-10-1 s-1
    • Figure 3: Anelastic strain (defined in Fig. 1) as a function of strain, for as-cast (a) AM40, (b) AM60, (c) AZ91, (d) AE44 and (e) T5-aged AE44, at strain rate range 10-6-10-1 s-1
    • Figure 4: EBSD maps of AE44 showing (a) twin-free microstructure in as-cast condition and the formation of different types of twins at (b,c) 10-6 s-1 and (d, e) 10-1 s-1 after cyclic testing to 3% strain.
    • Figure 5: TEM observations of dislocations pile-ups at twin and grain boundaries in AE44 cyclic tested to 3% strain at (a) 10-6 s-1 and (c) 10-1 s-1
    • Figure 6: A correlation between onsets of stages II and III with anelastic strain. (a) Monotonic tensile flow curve with the onsets of stage II marked by ‘O’ and stage III marked by ‘X’ and (b) stress as a function of anelastic strain at strain rate 10-4 s-1.
    • Figure 7: Maximum anelastic strain as a function of strain rate, for all alloys tested.
    • Figure 8: Offset strain (plastic strain + anelastic strain) as a function of permanent plastic strain for (a) AZ91 and (b) AE44 at strain rate range 10-6-10-1 s-1.
    • Figure 1: Monotonic (dashed) and cyclic (solid) stress-strain behaviour of (a) AZ91 and (b) AE44 at different strain rates, ε̇ =10-6-10-2 s-1
    • Figure 2: Applied stress as a function of Stage II strain, for as-cast (a) AM40, (b) AM60, (c) AZ91, (d) AE44 and (e) AE44-T5, at strain-rate range 10-6-10-1 s-1.
    • Figure 3: Comparison of Weibull function and power law.
    • Figure 4: Stress when εIII reaches 0.01 for as-cast AM40, AM60, AZ91, AE44 and T5-aged AE44 and 0.015 for AZ91 as a function of maximum anelastic stress.
    • Figure 5: Average deformation behaviour of stage II for AZ91 and AE44, best fit using Eq. (3) (dashed) are compared with experiments (solid).
    • Figure 6: Experimental flow curves (solid) of (a) AZ91 and (b) AE44 at strain rates 10-6-10-1 s-1 are compared with best fit linear lines (dashed) according to Eq. (4).
    • Figure 7: The strain hardening rate, Θ in Stage III for present alloys at different strain rates.
    • Figure 8: The prismatic yield stress, σy of present alloys at different strain rates.
    • Figure 9: Experimental (solid) and best fit (dashed) stress-strain curve of stage IV for (a) AZ91 and (b) AE44.
    • Figure 10: Comparison of Ludwik’s and Hollomon’s models with KIV and nIV of best fit of Eq. (13).
    • Figure 11: Comparison of experimental (coloured) and modelled (black) stress-strain curves of (a) AM40, (b) AM60, (c) AZ91, (d) AE44, and (e) AE44-T5 at wide strain-rate range 10-6-10-1 s-1
    • Figure 12: Enlarged stress-strain curve in the Stage II-III transition region for the present die-cast alloys.
    • Figure 8.2: Comparison of (a) proof stress and (b) % elongation to fracture of magnesium and aluminium casting alloys.
    • Figure 8.3: Fractions of (a) elastic and (b) anelastic + plastic for present die-cast magnesium alloys.
Fig. 3. Anelastic strain (defined in Fig. 1) as a function of strain, for as-cast (a) AM40, (b) AM60, (c) AZ91, (d) AE44 and (e) T5-aged AE44, at strain rate range 10−6 −10−1 s −1 . Onset of stage III, extensive prismatic slip (defined in Fig. 2) is marked by symbol ‘X’.
Fig. 3. Anelastic strain (defined in Fig. 1) as a function of strain, for as-cast (a) AM40, (b) AM60, (c) AZ91, (d) AE44 and (e) T5-aged AE44, at strain rate range 10−6 −10−1 s−1. Onset of stage III, extensive prismatic slip (defined in Fig. 2) is marked by symbol ‘X’.
Fig. 4. EBSD maps of AE44 showing (a) twin-free microstructure in as-cast condition and the formation of different types of twins at strain rates of (b, c) 10−6 s −1 and (d, e) 10−1 s −1 after cyclic testing to 3% strain. The loading direction is horizontal.
Fig. 4. EBSD maps of AE44 showing (a) twin-free microstructure in as-cast condition and the formation of different types of twins at strain rates of (b, c) 10−6 s−1 and (d, e) 10−1 s−1 after cyclic testing to 3% strain. The loading direction is horizontal.

6. 結論と考察:

  • 主な結果の要約: 本研究では、耐力測定、ひずみ速度感受性、アネラスティック性の矛盾を解決し、ダイカスト マグネシウム-アルミニウム合金の構成モデルを開発しました。より高いオフセットひずみ法は、耐力測定の一貫性を向上させました。ひずみ速度感受性は、α-マグネシウムマトリックス中のアルミニウム固溶体含有量の増加とともに低下しました。アネラスティック性はひずみ速度に鈍感であり、一方、 プリズムすべりによる塑性はひずみ速度に敏感でした。4 段階の構成モデルは、複雑な変形挙動を効果的に記述しました。
  • 研究の学術的意義: 本研究は、ダイカスト マグネシウム合金の耐力測定におけるより一貫性のある方法を提供し、材料特性の比較可能性を高めます。 底面すべりと可逆双晶が耐力に及ぼす影響を解明し、これらの合金の変形挙動を 4 つの明確な段階にわたって包括的に記述しています。この研究は、ひずみ速度感受性を緩和するアルミニウム固溶体の役割と、アネラスティック性を決定するすべりメカニズムと双晶メカニズムの複雑な相互作用を強調しています。
  • 実用的な意義: 提案された一貫性のある耐力測定法により、エンジニアリング用途におけるマグネシウム合金の特性をより信頼性の高い比較が可能になります。この知見は、マグネシウム合金、特に AE44 が、より高いひずみ速度でエネルギー吸収を向上させ、自動車構造用途における耐衝撃性に貢献できる可能性を示唆しています。
  • 研究の限界: 構成モデルは、AZ91 の第 II-III 遷移領域で応力をわずかに過大評価しており、高濃度合金のモデルをさらに改良する必要があることを示しています。

7. 今後のフォローアップ研究:

  • フォローアップ研究の方向性: 今後の研究では、アネラスティック性に対するひずみ速度とアルミニウム含有量の影響を、特に準静的ひずみ速度領域外でさらに探求する必要があります。変形メカニズム、特に析出物と金属間化合物ネットワークの役割に関するさらなる調査は、構成モデルを改良し、改良された構造用合金を開発するために必要です。
  • さらなる探求が必要な分野:
    • より高いひずみ速度におけるアネラスティック性に対するひずみ速度とアルミニウム含有量の影響
    • 析出物と金属間化合物ネットワークがアネラスティック性と変形挙動に及ぼす影響の正確なメカニズム
    • 特に AZ91 のような高アルミニウム含有量合金において、第 II-III 遷移領域におけるモデルの精度を向上させるための構成モデルの改良
    • AE44-T5 の疲労特性と、アネラスティック変形が疲労寿命に及ぼす影響の調査

8. 参考文献:

  • [1] M.A. Meyers, Dynamic Behaviour of Materials, John Wiley & Sons, New York, 1994.
  • [2] T.B. Abbott, M.A. Easton, R. Schmidt, Magnesium for crashworthy components, in: M. Byko (Ed.), Journal of The Minerals, Metals, and Materials Society, TMS Annual Meeting, California, 2003, pp. 227-230.
  • [2] C.A. Newland, M.T. Murray, Strain rate dependent behaviour of magnesium-based alloys, in: Proceedings of the First Australasian Congress on Applied Mechanics, Institution of Engineers, Australia, 1996, pp. 73-76.
  • [4] N. Stanford, M.R. Barnett, Effect of Al and Gd solutes on the strain rate sensitivity of magnesium alloys, Metall. Mater. Trans. A 41 (2010) 734-743.
  • [5] T.K. Aune, D. Albright, H. Westengen, T.E. Johnsen, B. Anderson, Behavior of die cast magnesium alloys subject to rapid deformation, SAE Technical Paper, 2000 pp. 2000-01- 1116.
  • [6] J.P. Weiler, J.T. Wood, Strain-rate effects of sand-cast and die-cast magnesium alloys under compressive loading, in: S.N. Mathaudhu, W.H. Sillekens, N.R. Neelameggham, N. Hort (Eds.), Magnesium Technology 2012, John Wiley & Sons, Florida, 2012, pp. 365-370.
  • [7] A.V. Nagasekhar, M.A. Easton, C.H. Cáceres, Solute content and the grain microstructure of high pressure die cast magnesium–aluminium alloys, Adv. Eng. Mater. 11 (2009). 912- 919.
  • [8] H.Q.Ang, S.M.Zhu, T.B.Abbott, Q.Dong, M.A.Easton, Strain-rate sensitivity of die-cast magnesium-aluminium based alloys
  • [Accepted]. http://dx.doi.org/10.1016/j.msea.2017.05.093
  • [9] H.Q. Ang, T.B. Abbott, S.M. Zhu, C.F. Gu, M.A. Easton, Proof stress measurement of diecast magnesium alloys, Mater. Des. 112 (2016) 402-409.
  • [10] E. Karimi, A. Zarei-Hanzaki, M.H. Pishbin, H.R. Abedi, P. Changizian, Instantaneous strain rate sensitivity of wrought AZ31 magnesium alloy, Mater. Des. 49 (2013) 173-180.
  • [11] W.S. Lee, T.H. Chen, Rate-dependent deformation and dislocation substructure of Al–Sc alloy, Scr. Mater. 54 (2006) 1463-1468.
  • [12] T. Matsunaga, H. Somekawa, H. Hongo, M. Tabuchi, Deformation mechanism transition with strain rate in Mg–3Al–1Zn alloy at room temperature, Mater. Sci. Eng. A 647 (2015) 212-215.
  • [13] R.B. Figueiredo, F. Poggiali, C. Silva, P.R. Cetlin, T.G. Langdon, The influence of grain size and strain rate on the mechanical behavior of pure magnesium, J. Mater. Sci. 51 (2016). 3012-3024.
  • [14] X.Z. Lin, D.L. Chen, Strain hardening and strain-rate sensitivity of an extruded magnesium alloy, J. Mater. Eng. Perform. 17 (2008). 894-901.
  • [15] L.P. Kubin, Y. Estrin, Dynamic strain ageing and the mechanical response of alloys, J. Phys. III 1 (1991) 929-943.
  • [16] L. Jiang, J.J. Jonas, R. Mishra, Effect of dynamic strain aging on the appearance of the rare earth texture component in magnesium alloys, Mater. Sci. Eng. A 528 (2011) 6596-6605.
  • [17] A. Van den Beukel, Theory of the effect of dynamic strain aging on mechanical properties, Phys. Stat. Sol. (a) 30 (1975) 197-206.
  • [18] Z. Trojanová, P. Lukác, K.U. Kainer, V. Gärtnerová, Dynamic strain ageing during stress relaxation in selected magnesium alloys containing rare earth elements, Adv. Eng. Mater. 7 (2005) 1027-1032.
  • [19] S.M. Zhu, J.F. Nie, M.A. Gibson, M.A. Easton, P. Bakke, Microstructure and creep behavior of high-pressure die-cast magnesium alloy AE44, Metall. Mater. Trans. A 43 (2012) 4137-4144.
  • [20] S.M. Zhu, M.A. Easton, T.B. Abbott, M.A. Gibson, J.F. Nie, The influence of individual rare earth elements (La, Ce, or Nd) on creep resistance of die‐cast magnesium alloy AE44, Adv. Eng. Mater. 18 (2016) 932-937.
  • [21] M.I. Khan, A.O. Mostafa, M. Aljarrah, E. Essadiqi, M. Medraj, Influence of cooling rate on microsegregation behavior of magnesium alloys, J. Mater. (2014) 1-18.

9. 著作権:

この資料は上記の論文に基づいて要約されたものであり、商業目的での無断使用は禁止されています。
Copyright © 2025 CASTMAN. All rights reserved.

PDF View

Tags: A380; ,Applications; ,CAD; ,Die casting; ,Efficiency; ,Magnesium alloys; ,Microstructure; ,Review; ,자동차; ,해석